Congruence
This lesson discusses basic terms for geometry. Many polygons have the property of lines of symmetry, or rotational symmetry. Rotations, reflections, and translations are ways to create congruent polygons.
Geometry Terms
The terms point, line, and plane help define other terms in geometry. A point is an exact location in space with no size and has a label with a capital letter. A line has location and direction, is always straight, and has infinitely many points that extend in both directions. A plane has infinitely many intersecting lines that extend forever in all directions.

The diagram shows point W, point X, point Y, and point Z. The line is labeled as \(\overleftrightarrow{WX}\) , and the plane is Plane A or Plane WYZ (or any three points in the plane).
With these definitions, many other geometry terms can be defined. Collinear is a term for points that lie on the same line, and coplanar is a term for points and/or lines within the same plane. A line segment is a part of a line with two endpoints. For example, \(\overline{WX }\) has endpoints W and X. A ray has an endpoint and extends forever in one direction. For example, \(\overrightarrow{ AB}\) has an endpoint of A, and \(\overrightarrow{ BA}\) has an endpoint of B. The intersection of lines, planes, segment, or rays is a point or a set of points.
Some key statements that are evident in geometry are:
- There is exactly one straight line through any two points.
- There is exactly one plane that contains any three non-collinear points.
- A line with points in the plane lies in the plane.
- Two lines intersect at a point.
- Two planes intersect at a line.
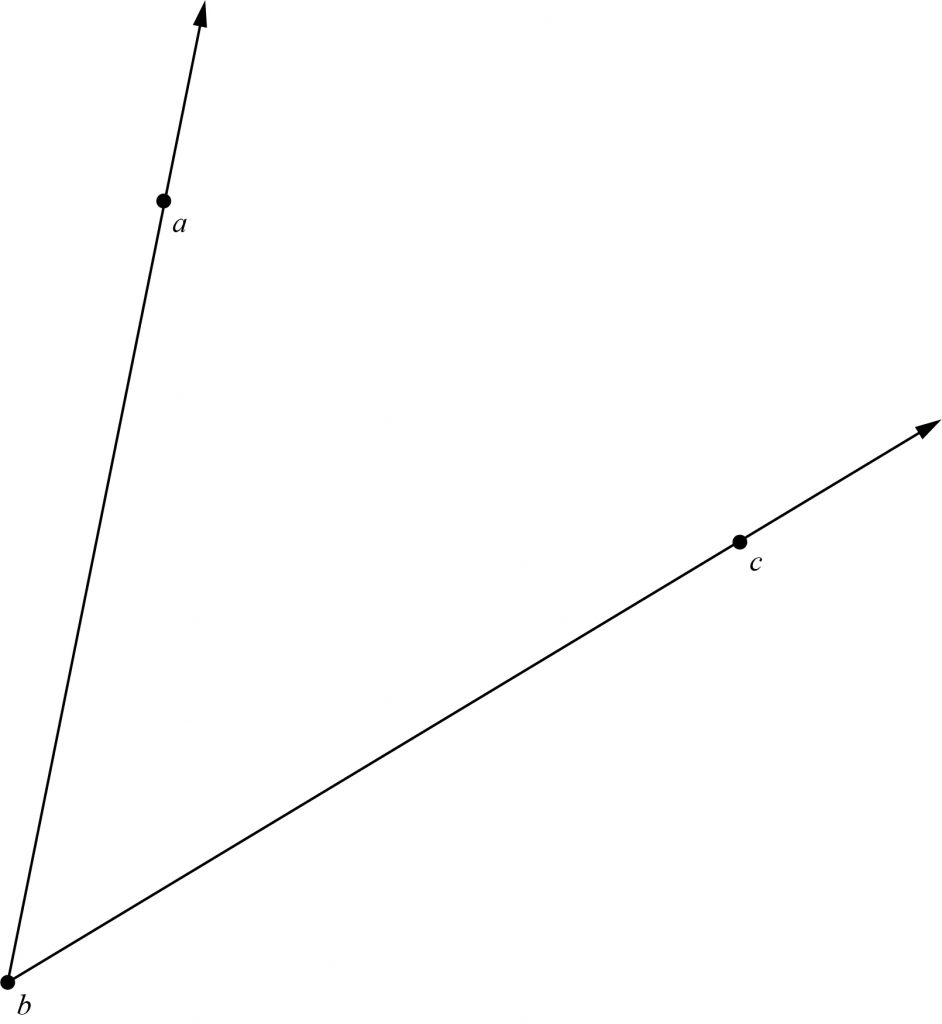
Two rays that share an endpoint form an angle. The vertex is the common endpoint of the two rays that form an angle. When naming an angle, the vertex is the center point. The angle below is named ∠ABC or ∠CBA.
An acute angle has a measure between 0° and 90°, and a 90° angle is a right angle. An obtuse angle has a measure between 90° and 180°, and a 180° angle is a straight angle.
There are two special sets of lines. Parallel lines are at least two lines that never intersect within the same plane. Perpendicular lines intersect at one point and form four angles.

BE CAREFUL!
Lines are always named with two points, a plane can be named with three points, and an angle is named with the vertex as the center point.
Example
Geometry Terms Review
Line and Rotational Symmetry
Symmetry is a reflection or rotation of a shape that allows that shape to be carried onto itself. Line symmetry, or reflection symmetry, is when two halves of a shape are reflected onto each other across a line. A shape may have none, one, or several lines of symmetry. A kite has one line of symmetry, and a scalene triangle has no lines of symmetry.

Rotational symmetry is when a figure can be mapped onto itself by a rotation about a point through any angle between 0° and 360°. The order of rotational symmetry is the number of times the object can be rotated. If there is no rotational symmetry, the order is 1 because the object can only be rotated 360° to map the figure onto itself. A square has 90° rotational symmetry and is order 4 because it can be rotated 90°, 180°, 270°, and 360°. A trapezoid has no rotational symmetry and is order 1 because it can only be rotated 360° to map onto itself.
KEEP IN MIND

A polygon can have both, neither, or either reflection and rotational symmetry.
Example
Line and Rotational Symmetry Review
Rotations, Reflections, and Translations
There are three types of transformations: rotations, reflections, and translations. A rotation is a turn of a figure about a point in a given direction. A reflection is a flip over a line of symmetry, and a translation is a slide horizontally, vertically, or both. Each of these transformations produces a congruent image.
A rotation changes ordered pairs (x, y) in the coordinate plane. A 90° rotation counterclockwise about the point becomes (–y, x), a 180° rotation counterclockwise about the point becomes (–x, –y), and a 270° rotation the point becomes (y, –x). Using the point (6, –8):
- 90° rotation counterclockwise about the origin (8, 6)
- 180° rotation counterclockwise about the origin (–6, 8)
- 270° rotation counterclockwise about the origin (–8, –6)
A reflection also changes ordered pairs (x, y) in the coordinate plane. A reflection across the x-axis changes the sign of the y-coordinate, and a reflection across the y-axis changes the sign of the x-coordinate. A reflection over the line y = x changes the points to (y, x), and a reflection over the line y = –x changes the points to (–y, –x). Using the point (6, –8):
- A reflection across the x-axis (6, 8)
- A reflection across the y-axis (–6, –8)
- A reflection over the line y = x (–8, 6)
- A reflection over the line y = –x (8, –6)
A translation changes ordered pairs (x, y) left or right and/or up or down. Adding a positive value to an x-coordinate is a translation to the right, and adding a negative value to an x-coordinate is a translation to the left. Adding a positive value to a y-coordinate is a translation up, and adding a negative value to a y-coordinate is a translation down. Using the point (6, –8):
- A translation of (x + 3) is a translation right 3 units (9, –8)
- A translation of (x – 3) is a translation left 3 units (3, –8)
- A translation of (y + 3) is a translation up 3 units (6, –5)
- A translation of (y – 3) is a translation down 3 units (6, –11)

KEEP IN MIND
A rotation is a turn, a reflection is a flip, and a translation is a slide.
Example
Rotations, Reflections, and Translations Review
Let’s Review!
- The terms point, line, and plane help define many terms in geometry.
- Symmetry allows a figure to carry its shape onto itself. This can be reflectional or rotational symmetry.
- Three transformations are rotation (turn), reflection (flip), and translation (slide).
Subscribe to the online course to gain access to the full lesson content.
If your not ready for a subscription yet, be sure to check out our free practice tests and sample lesson at this link
