Circles
TEAS Test – Circles
In the previous lesson you learned the TEAS exam math topic of similarity, right triangles and trigonometry. This lesson introduces the TEAS exam math topic of circles, including finding the circumference and the area of the circle.
TEAS Test – Circles Terminology
A circle is a figure composed of points that are equidistant from a given point. The center is the point from which all points are equidistant. A chord is a segment whose endpoints are on the circle, and the diameter is a chord that goes through the center of the circle. The radius is a segment with one endpoint at the center of the circle and one endpoint on the circle. Arcs have two endpoints on the circle and all points on a circle between those endpoint.
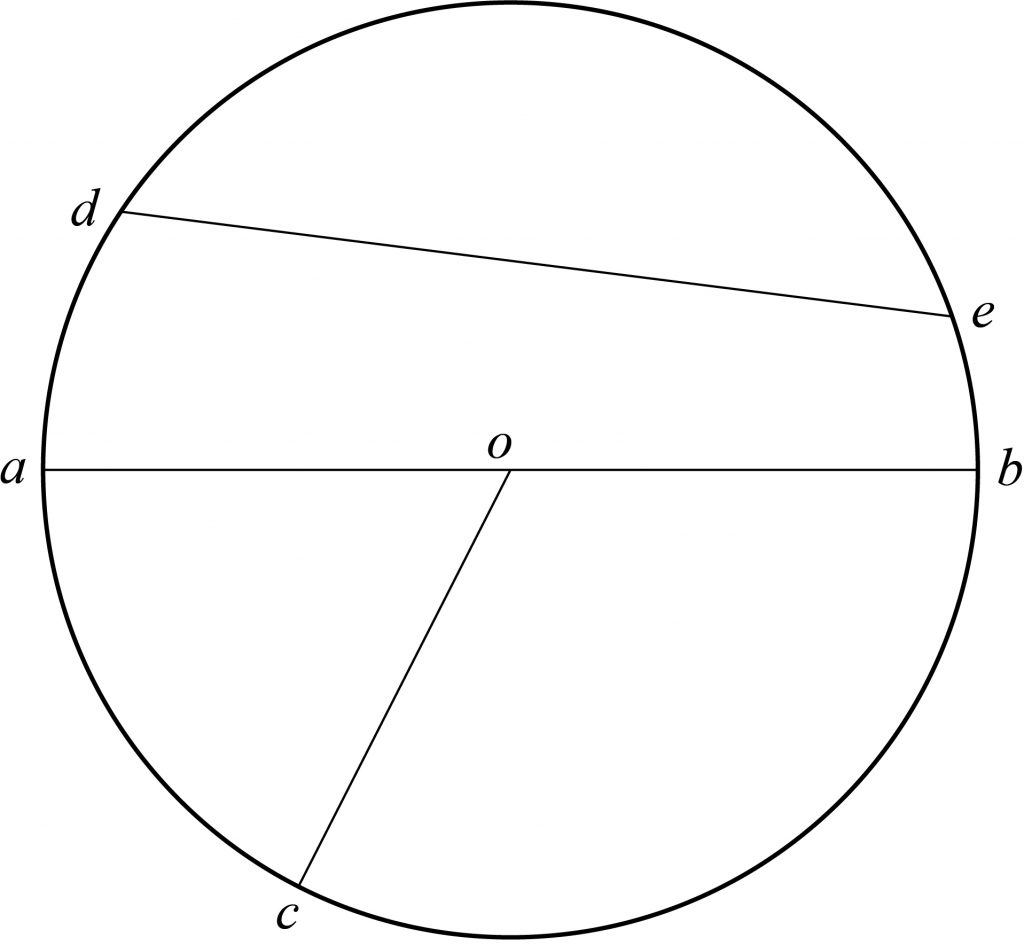
In the circle at the right, O is the center, \(\overline{OC}\) is the radius, \(\overline{AB}\) is the diameter, \(\overline{DE}\) is a chord, and \(\hat{AD}\) is an arc.

Keep In Mind
The radius is one-half the length of the diameter of the circle.
Try this TEAS sample math question
Circle Terminology Review
TEAS Test – Circumference and Area of a Circle
The circumference of a circle is the perimeter, or the distance, around the circle. There are two ways to find the circumference. The formulas are the product of the diameter and pi or the product of twice the radius and pi. In symbol form, the formulas are C = πd or C = 2πr.
The area of a circle is the amount of space inside a circle. The formula is the product of pi and the radius squared. In symbol form, the formula is \(A = \pi r^2\). The area is always expressed in square units.
Given the circumference or the area of a circle, the radius and the diameter can be determined. The given measurement is substituted into the appropriate formula. Then, the equation is solved for the radius or the diameter.
Be Careful

Make sure that you apply the correct formula for circumference and area of a circle.
Try these TEAS sample math questions
Circumference and Area of a Circle Review
TEAS Test – Finding Circumference or Area Given the Other Value
Given the circumference of a circle, the area of the circle can be found. First, substitute the circumference into the formula and find the radius. Substitute the radius into the area formula and simplify.
Reverse the process to find the circumference given the area. First, substitute the area into the area formula and find the radius. Substitute the radius into the circumference formula and simplify.
Be Careful

Pay attention to the details with each formula and apply them in the correct order.
Try these TEAS sample math questions
Finding Circumference or Area Given the Other Value Review
Let’s Review!
- Key terms related to circles are radius, diameter, chord, and arc. Note that the diameter is twice the radius.
- The circumference or the perimeter of a circle is the product of pi and the diameter or twice the radius and pi.
- The area of the circle is the product of pi and the radius squared.
In the next lesson we move on to Algebra and discuss the TEAS math test topic of equations with one variable.
You May Subscribe to the online course to gain access to the full lesson content.
If your not ready for a subscription yet, be sure to check out our free practice tests and sample lesson at this link
