Electricity and Magnetism

Magnetism manifests through forces and fields in a manner similar to electricity, but the mathematics are more complicated. Qualitatively, a simple model of magnetism is relatively easy to understand. Magnetic fields and magnetic forces arise from moving charges—that is, any charged object with a nonzero velocity produces a magnetic field (and thus can exert a magnetic force on another moving charge). For instance, a wire that carries an electric current—which is the movement of negatively charged electrons through the wire—creates a magnetic field around that wire. The movement of electrons around the nucleus of an atom also creates a magnetic field, and in some elements (such as iron), the result can be powerful magnetic properties. Earth has a magnetic field that allows navigation using a compass, which uses a small magnetic needle to detect the direction of the field.

Like electric charge, magnetism has two “polarities” (or poles) called north and south. Unlike electric charge, however, a magnetic object (or magnet) always has a magnetic north and a magnetic south—north and south never exist by themselves. (Positive and negative electric charges can exist by themselves.) In addition, like polarities repel, and unlike polarities attract.

---

BE CAREFUL!

Remember that any motion of charge creates a magnetic field, but only charge acceleration creates electromagnetic waves.

---

DID YOU KNOW!

---

Because electric currents create magnetic fields, they can deflect a compass needle. For instance, if you connect a wire across the terminals of a battery, causing an electric current to flow, you can see the effect of magnetism if you bring a compass near it. An accidental observation of this phenomenon led to the discovery of the link between electric current and magnetism.

---

Electric and Magnetic Flux

Electric flux is the “flow” of the electric field through a given surface. To envision this concept, drawing electric field lines is helpful. Field lines show the direction of the electric force in space—specifically, the path a positive “test charge” would follow if it were initially stationary at some arbitrary point in space. By convention, field lines are generally shown flowing out from positive charges and in to negative charges. The illustration below shows a positive charge in empty space, a negative charge in empty space, and a positive and negative charge in close proximity.

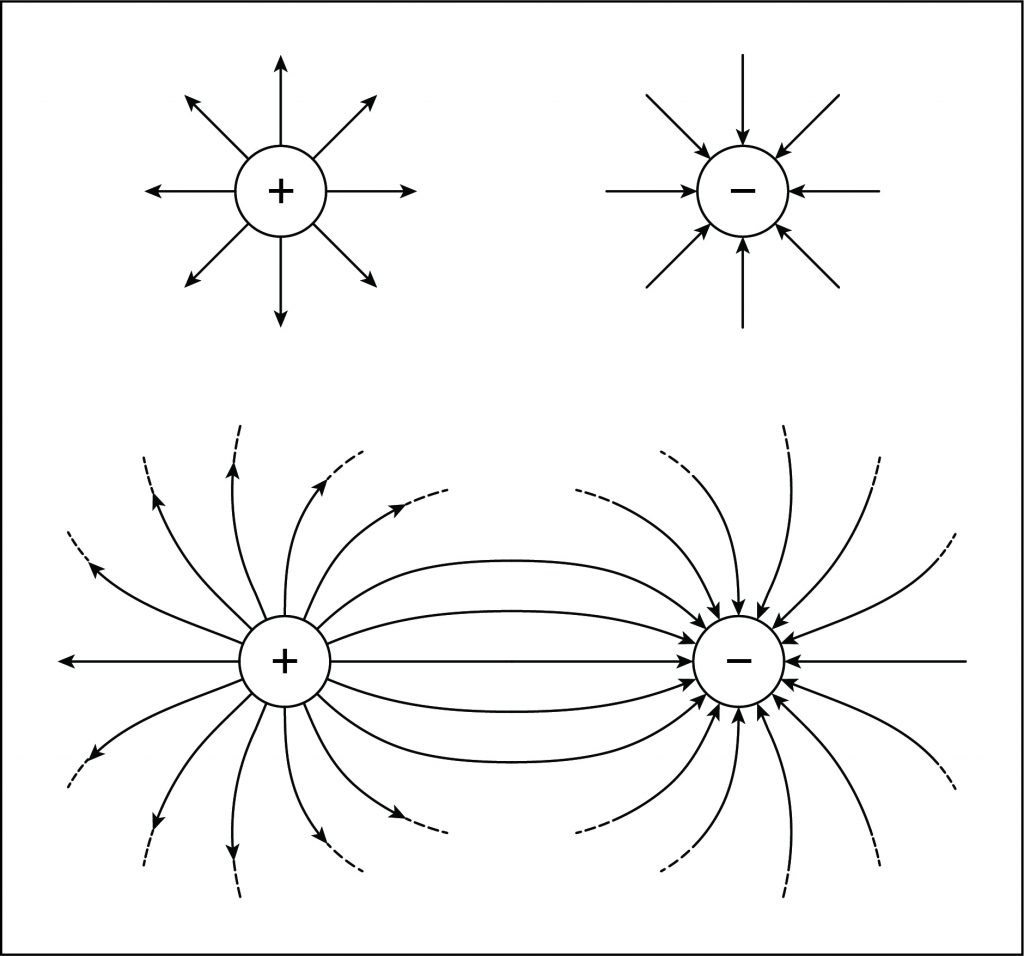
The more field lines that flow through an area, the greater the flux. Higher field-line density indicates a stronger field or force in that region. Although field lines are conceptual rather than physical, they are a helpful way to represent how electricity and magnetism permeate the space around charged objects.

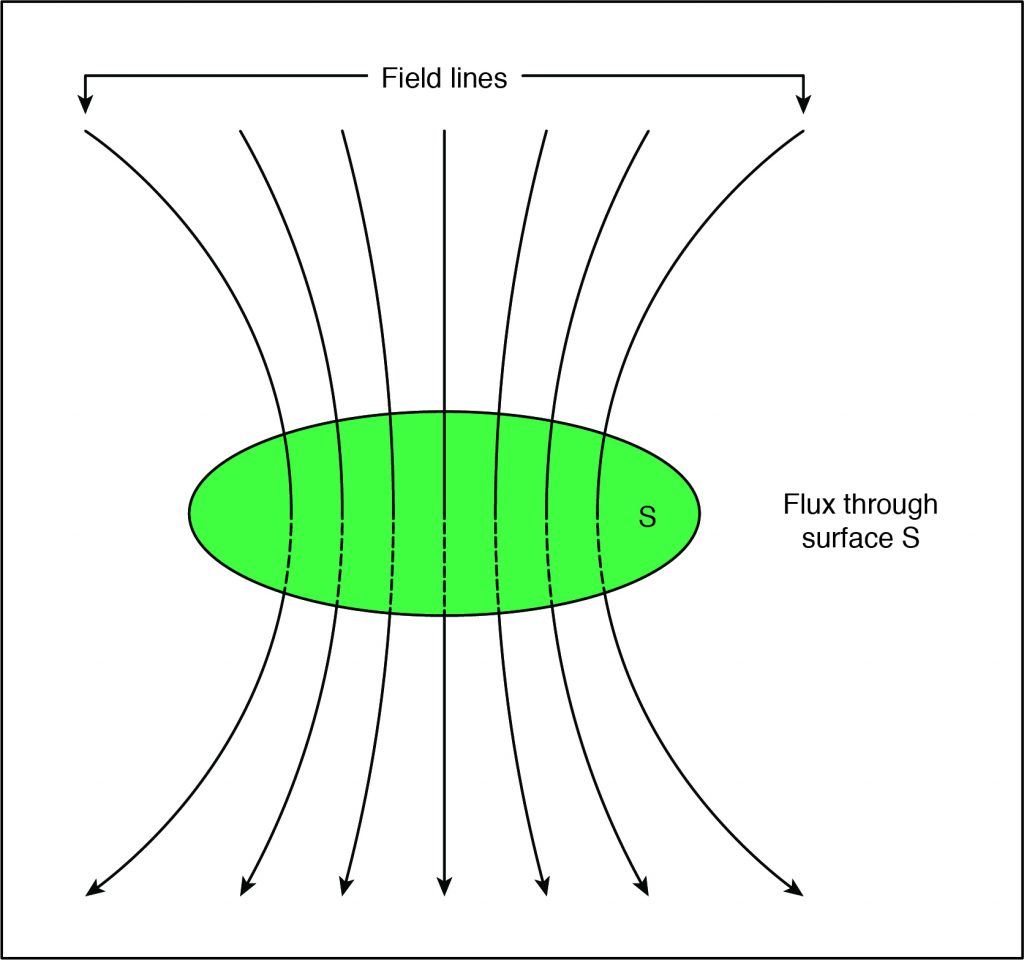
Magnetic flux and magnetic field lines are analogous to electric flux and field lines, but they represent magnetism rather than electricity. The same general principles apply.

One aspect of electricity and magnetism underlying much of today’s technology is electromagnetic induction. This phenomenon occurs when an electrical conductor such as a wire experiences a changing magnetic field: the result is an electric force in that conductor. The strength of the electric force around a conducting loop is proportional to the rate at which the magnetic flux through that loop is changing. For example, spinning a coil of wire positioned between powerful magnets (or vice versa) is essentially how power companies produce electricity. Electrically driven motors apply the same principles, but in reverse.

Electric Circuits

Electric circuits are a critical component of many modern technologies, including computing technology and electrical power distribution. An electric circuit (or just circuit) is a closed “loop” in which electric charge experiences an electric force around the loop. Important parameters in a circuit are voltage, current, and resistance. Voltage, also called the electric potential difference, is the amount of energy required to move a unit of charge between two points in a circuit. Current is the amount of charge flowing through a given surface per second. Resistance is a measure of how much an electrical component impedes the flow of current.

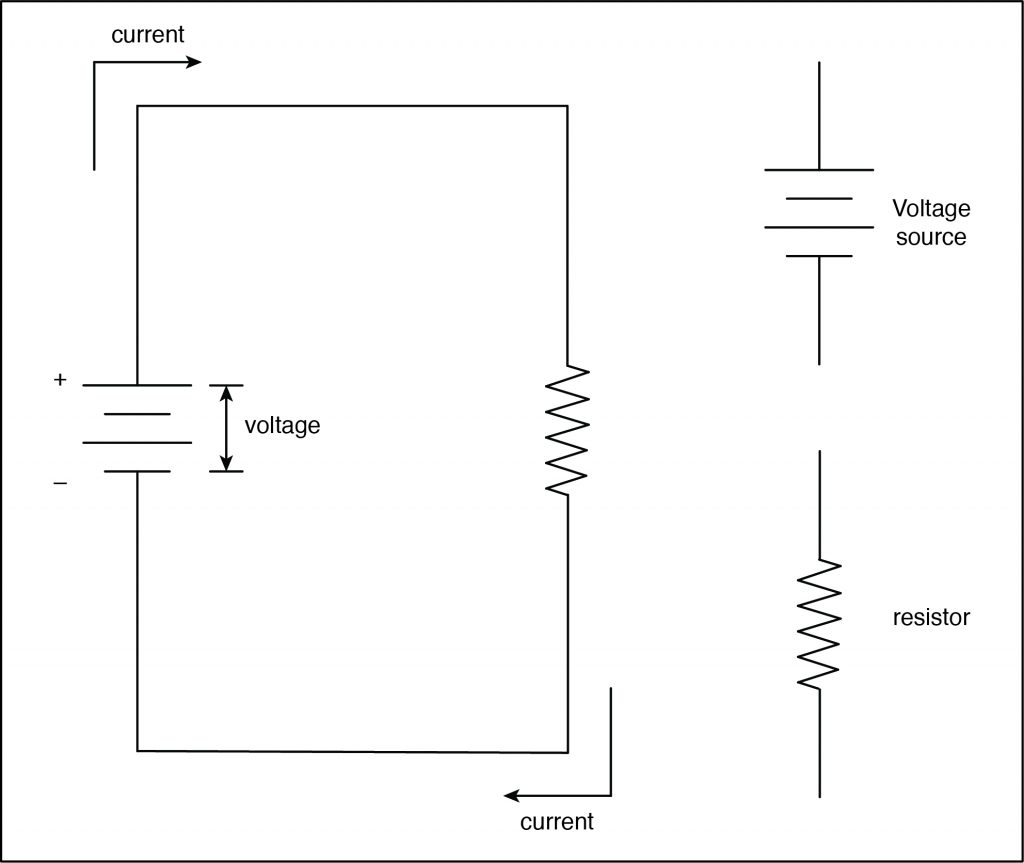
A simple example of a circuit includes a voltage source (for example, a battery) and a resistor (for example, a light bulb) connected by metal wires. A simple voltage source has two terminals: it maintains an electric potential difference across those terminals so that charge will try to flow from the higher-voltage (“positive”) terminal to the lower-voltage (“negative”) terminal.

Voltage is usually measured in volts (V); current is usually measured in amperes (A) or amps, which are coulombs per second; and resistance is usually measured in ohms (Ω). Materials, such as metal, that allow the free flow of electric charge are called conductors. Materials, such as many plastics, that do not  allow the free flow of electric charge are called insulators.

DID YOU KNOW?

---

By convention, current is defined as the flow of positive charge. But because negative  charge (electrons) is what actually flows in basic circuits, the mathematical assignment of a negative sign to the electron’s charge created a historical dilemma. Mathematically, the flow of positive charge in one direction is equal to the flow of negative charge in the opposite direction, so the common practice is to discuss the flow of positive charge even though electrons often constitute the current.

---

Ohm’s Law

Circuit analysis can become extremely complex when the circuit involves many components, but a ew basic principles can aid the process, especially for simple circuits. One example is Ohm’s law, which relates the current I  through and voltage V  across a component with a given resistance R: V = IR.

FOR EXAMPLE

---

If a circuit involves a 10-volt battery connected to a 100-ohm resistor, you can find the current through the resistor using Ohm’s law:

V=IR

\(I=\frac{V}{R}=\frac{10hspace{0.05mm}volts}{100hspace{0.05mm}ohms}= 0.1hspace{0.05mm}amps\)

---

Two important rules also help in analyzing circuits. The first rule is that at any point (or node) in the circuit, the current flowing into that point must equal the current flowing out of that point. Thus, if three wires join at a node, the sum of the currents flowing in must equal the sum of the currents flowing out. The second rule is that for any closed loop in the circuit, the sum of the voltages around the loop must equal zero. For this rule, going around the entire loop in the same direction is critical. One convention is that going from a higher voltage to a lower voltage represents a positive voltage change (or voltage “drop”), whereas going from a lower voltage to a higher voltage represents a negative voltage change. Thus, in a circuit containing just a battery and a resistor, the voltage change across the resistor must be equal in magnitude but opposite in sign to the voltage change across the battery (when going either clockwise or counterclockwise around the circuit).

Let’s Review!

• Electric charges, which can be either positive or negative, create electric fields and exert an electric force on other charges.
• The electric force between two charges (\(Q_1\) and \(Q_2\)) obeys Coulomb’s law \(F_E=kfrac{Q_1Q_2}{r^2}\) where k  is the electric constant (about \(9 × 10^9\) when working in SI units) and r is the distance between the charges.
• The electric field from a charge Q is \(E=kfrac{Q}{r^2}\).
• Magnetic fields and forces result from moving charges (an electric current). Magnets have north and south polarities, but unlike electricity where negative and positive charges can appear separately, these magnetic polarities always appear together.
• Electric flux is the “flow” of the electric field, which can be visualized using electric field lines.
• Magnetic flux is the “flow” of the magnetic field, which can be visualized using magnetic field lines.
• Changing magnetic flux through a surface creates an electric field through that surface—a phenomenon that enables electricity generation.
• Electric circuits involve current flowing through resistors and other electrical components, driven by an electric potential difference (voltage).
• The voltage across a resistor is equal to the product of the resistance and the current (Ohm’s law). Common units for these parameters are volts, amperes, and ohms.